4. Human Capital

KAT.TAL.322 Advanced Course in Labour Economics

Nurfatima Jandarova

March 14, 2024

Today

• Overview
• Productive human capital investments
• Signalling theory
• Returns to education

Human capital

Labour heterogeneity is important for labour supply and demand.

Human capital includes education, training, health investments.

First references as early as Adam Smith; formalised by Becker in 1960s.

Overview

Overview

Human capital is an investment

• benefit: gain in earnings
• cost: tuition, foregone earnings, psychological costs

Two main camps for source of gain in earnings:

• gain in productivity

• signalling

Human capital production function

Typically, univariate (years of education), but can be complex function of

• Innate skills (e.g., genetics)

• Parental investments (e.g., day care, time spent with children, tutors)

• Schooling/formal education

  • Quantity (e.g., high school vs university)
  • School quality (e.g., teacher quality, expenditure per student)
  • Differences in curricula/fields (e.g., STEM vs arts)

• Peers (e.g., at school, at work)

• On-the-job training (e.g., general vs specific skills)

In itself, entire field of research.

Covered in course: investment decisions, years of education, a little of quality next lecture

See Cunha and Heckman (2007) for a nice framework of pre-market production of skills

Productive human capital investments

Basic model

Assume education choice \(S \in \{HS, C\}\)

Worker with \(s\) produces \(Y_s\) goods when employed by a firm, \(\forall s \in S\).

Perfect competition ensures that \(W_{HS} = Y_{HS}\) and \(W_C = Y_C\)

Assume cost of education given by function \(\eta(S)\)

Then choose college if marginal benefit outweighs marginal cost

\[ S = C \iff \color{#288393}{W_{C} - W_{HS}} \geq \color{#9a2515}{\eta(C) - \eta(HS)} \]

Static (single-period) model

• earnings are perfectly summarised by \(Y_s\) (could be DPV of earnings)

• can also make it more interesting by adding individual heterogeneity

• people invest into education because productivity \(\uparrow\) which is correctly priced by the market

  • if there are market failures, then typically social benefits > individual benefits.

  • For example, monopsony pays wages below marginal product of labour.

  • Or there may be other benefits to education, that market doesn’t price (low criminality, better peers)

  • In these cases, people under-educate themselves

• single decision at the beginning, no dynamics

Lifecycle model: simplified Ben-Porath (1967)

• Divide time between schooling/training \(\sigma(t)\) and working \(1 - \sigma(t)\)
• Law of motion of HC: \(\dot{h}(t) = \theta \sigma(t)h(t)\)
• Production function per worker: \(y(t) = Ah(t)\) = wage
• Assume linear utility and no utility cost of \(\sigma(t)\)

\[ \Omega = \int_0^T \left(1 - \sigma(t)\right) Ah(t)e^{-rt} dt \qquad \text{s.t. HC law of motion} \]

Marginal return to HC effort \(\sigma(t)\) is

\(\frac{\partial \Omega}{\partial \sigma(t)} = -Ah(t)e^{-rt} + \int_0^T \left(1 - \sigma(z)\right) A\frac{\partial h(z)}{\partial \sigma(t)} e^{-rz} dz\)

\(\frac{\partial \Omega}{\partial \sigma(t)} = \color{#8e2f1f}{\underbrace{-Ah(t)e^{-rt}}_\text{foregone earnings}} + \color{#288393}{\underbrace{A\theta\int_t^T \left(1 - \sigma(z)\right) h(z) e^{-rz} dz}_\text{discounted future payoff}}\)

• Walk-through the HC law of motion (meaning)!

• No utility cost means only opportunity cost matters for the decision!

• Walk through the “derivation” of \(\Omega\) (implies linear utility)

Law of motion of HC

\[ \begin{align*} \frac{d h(t)}{dt} &= \theta \sigma(t) h(t) \Rightarrow \\ \frac{d \ln h(t)}{dt} &= \theta \sigma(t) \Rightarrow \\ \ln h(t) - \ln h(0) &= \int_0^t \frac{d\ln h(z)}{dz}dz = \int_0^t \theta \sigma(z)dz \Rightarrow \\ h(t) &= h(0) \exp\left(\theta \int_0^t \sigma(z)dz\right) \end{align*} \]

Thus, the term we need to figure out for the FOC is \(\frac{\partial h(z)}{\partial \sigma(t)}\). The first part is easy: the derivative is equal to zero whenever \(z < t\) because the accumulated human capital is not yet a function of effort at time \(t\). The second part when \(z \geq t\) is trickier:

\[ \begin{align*} \frac{\partial h(z)}{\partial \sigma(t)} &= \frac{\partial}{\partial \sigma(t)} \left(h(0)\exp\left[\theta \int_0^z \sigma(s)ds\right]\right) = \\ &= h(0)\exp\left[\theta \int_0^z \sigma(s)ds\right] \theta \frac{\partial}{\partial \sigma(t)} \left(\int_0^z \sigma(s)ds\right) = \\ &= h(z) \theta \frac{\partial}{\partial \sigma(t)} \left(\int_0^z \sigma(s)ds\right) \end{align*} \]

The last term is a functional derivative of a function:

\[ \begin{align*} V[\sigma] &= \int \sigma(r) dr \Rightarrow \\ \int\frac{\delta V}{\delta \sigma(r)} \phi(r)dr &= \left[\frac{d}{d\varepsilon} \int \sigma(r) + \varepsilon \phi(r) dr\right]_{\varepsilon = 0} = \\ &= \int \phi(r) dr \Rightarrow \\ \frac{\delta V}{\delta \sigma(r)} = 1 \end{align*} \]

Hence, the last term is equal to 1 and \(\frac{\partial h(z)}{\partial \sigma(t)} = \theta h(z)\) if \(z \geq t\).

Lifecycle model: simplified Ben-Porath (1967)

Optimal effort is zero at low efficiency \(\theta\) and high discount rate \(r\)

The change in marginal return over time is given by

\[ \frac{d}{dt}\left(\frac{\partial \Omega}{\partial \sigma(t)}\right) = A h(t) e^{-rt}(r - \theta) \]

If \(r > \theta\), then marginal return \(\uparrow\) over time, but is negative at \(T\):

\[ \frac{\partial \Omega}{\partial \sigma(T)} = -Ah(T)e^{-rT} < 0 \]

Hence, marginal return at every period is negative \(\Rightarrow \sigma^*(t) = 0 \quad \forall t\).

Lifecycle model: simplified Ben-Porath (1967)

Optimal effort when efficiency \(\theta\) is high or discount rate \(r\) is low

Marginal return \(\downarrow\) over time \(\Rightarrow\) may exist \(t = s\) such that \(\frac{\partial \Omega}{\sigma(s)} = 0\)

• initial investment into education \(\sigma^*(t) = 1, \quad \forall t \leq s\)
• work rest of the time \(\sigma^*(t) = 0, \quad \forall t > s\)
• study longer if \(\theta\) higher
  \[s = \begin{cases}T + \frac{1}{r}\ln\left(\frac{\theta - r}{\theta}\right) & \text{if } \theta \geq \frac{r}{1 - e^{-rT}} \\ 0 & \text{otherwise}\end{cases}\]

Can draw by hand to show that \(s\) is increasing in \(\theta\)

Lifecycle model: Ben-Porath (1967)

Allows for human-capital depreciation and on-the-job training

Source: Figure 4.9 from Cahuc (2004)

Draw by hand second curve for higher \(\theta\)

Highlight again that \(h(t)\) directly affects actual productivity of workers in these models

Signalling theory

Basic model

• Two types of productivity \(\theta_H\) and \(\theta_L\)
• Education \(e\) costs \(c_i = \frac{e}{\theta_i}\)
• Linear utility \(w - c_i, ~ \forall i \in \{H, L\}\)

Observable types

Free entry ensure \(w = \theta_i \Rightarrow e_i^* = 0, ~\forall i \in \{H, L\}\)

Unobservable types

• Low type gets no education \(e_L^* = 0\) and a payoff \(\theta_L\)
• High type gets \(e_H^* = \theta_L\left(\theta_H - \theta_L\right)\) and a payoff \(\theta_H - \frac{\theta_L\left(\theta_H - \theta_L\right)}{\theta_H}\)

• Walk through the separating solution before showing it!

• Productive theory suggests that people under-educate themselves because of positive externalities

• Signalling theory, however, says people overeducate themselves! Since education does nothing to productivity, it is only an annoying cost that people have to put up with.

• Hence, people over-educate themselves!

Solution steps:

1. In a separating equilibrium, the types do not want to pretend to be of other types
   

   \[\begin{cases} \theta_H - \frac{e_H}{\theta_H} \geq \theta_L - \frac{e_L}{\theta_H} \\ \theta_L - \frac{e_L}{\theta_L} \geq \theta_H - \frac{e_H}{\theta_L} \end{cases}\]

   
   \(\Rightarrow \theta_L \left(\theta_H - \theta_L\right) \leq e_H - e_L \leq \theta_H \left(\theta_H - \theta_L\right)\)

2. Given that costs \(c_L\) satisfies that above condition, we can find \(e_L^*\) that maximises individual payoff. This is easy, \(e_L^* = 0\) and her payoff is exactly \(\theta_L\).

3. Given the separation condition, the optimal education level of high type is \(e_H^* = \theta_L \left(\theta_H - \theta_L\right)\) and her payoff is \(\theta_H - \frac{\theta_L\left(\theta_H - \theta_L\right)}{\theta_H}\).

Returns to education

J. Mincer (1958)

• \(E(S)\) earnings with \(S\) years of schooling
• Assume no direct cost of education
• Internal rate of return: \(r\) that equates costs and benefits

Present value of earnings \(P(S) = \int_S^T E(S) e^{-rt} dt = E(S) \frac{e^{-rS} - e^{-rT}}{r}\)

\[ P(S) = P(0) \Rightarrow \ln E(S) \approx \ln E(0) + rS \]

Source: J. A. Mincer (1974), Table 5.1

Regression	\(R^2\)
\(\ln w = 7.58 + 0.070 S\)	0.067

• Linear utility, no direct cost, no heterogeneity => can estimate actual returns (net of costs = 0)

• Otherwise, the estimates only show “revenue” side of story.

• walk-through the derivation

\[ \begin{align} P(0) &= E(0) \frac{1 - e^{-rT}}{r}\\ P(0) = P(S) &\Rightarrow E(0) \frac{1 - e^{-rT}}{r} = E(S) \frac{e^{-rS} - e^{-rT}}{r}\\ E(0)\left(1 - e^{-rT}\right) &= E(S)\left(e^{-rS} - e^{-rT}\right) \\ E(S) &= E(0) \frac{1 - e^{-rT}}{e^{-rS} - e^{-rT}} \\ E(S) &= E(0) e^{rS}\frac{1 - e^{-rT}}{1 - e^{-r(T - S)}} \\ \ln E(S) &= \ln E(0) + rS + \ln\left(\frac{1 - e^{-rT}}{1 - e^{-r(T - S)}}\right) \end{align} \]

• what about life-cycle, experience (next slide)

J. A. Mincer (1974)

Accounting for experience

Building on Ben-Porath (1967)

• \(t(x)\) share of time dedicated to training at \(x\) experience and \(s\)
• HC law of motion: \(\dot{h}(s + x) = \rho_1 t(x)h(s + x), ~ \forall x \in [0, T - s]\)

\[\ln w(s + x) = \ln w(0) + \rho s + \rho_1 t(0) x - \rho_1\frac{t(0)}{2T} x^2\]

Source: J. A. Mincer (1974), Table 5.1

Regression	\(R^2\)
\(\ln w = 6.20 + 0.107 S + 0.081 X - 0.0012 X^2\)	0.285

Steps:

1. Integrate HC law of motion: \(h(s + x) = h(s) e^{\rho_1\int_0^x t(v)dv}\)
2. Assume again that \(w(t) = Ah(t)\); then
   \[ \begin{align} w(s + x) &= Ah(s)e^{\rho_1 \int_0^x t(v)dv} = w(s) e^{\rho_1 \int_0^x t(v)dv}\\ \ln w(s + x) &= \ln w(s) + \rho_1\int_0^x t(v)dv \end{align} \]
3. Assume linear decay of training function: \(t(x) = t(0)\left(1 - \frac{x}{T}\right)\). Then
   \[ \int_0^x t(0)\left(1 - \frac{v}{T}\right)dv = t(0)x - \frac{t(0)}{2T}x^2 \]
4. Plug in the above and \(\ln w(s) = \ln w(0) + \rho s\) into the expression in point 2
   \[\ln w(s + x) = \ln w(0) + \rho s + \rho_1 t(0) x - \rho_1\frac{t(0)}{2T} x^2\]

Notes:

• Parameter \(\rho_1\) can be interepreted as the rate of return to on-the-job training
• Estimate of \(\rho \uparrow\)
  • First regression suffers from omitted variable bias
  • Experience and schooling are negatively correlated

OLS estimates of returns to schooling

Potential issues

• Endogeneity of schooling and earnings

  • Cognitive and noncognitive abilities (Heckman, Stixrud, and Urzua 2006)

• Return to education is same regardless of duration of study

• Does not take into account direct costs of education

• Heterogeneity of returns (e.g., family background, schooling system)

• Years of schooling vs qualifications

• Productivity vs signalling interpretation

More on these in Card (1999)

Causal estimates of returns to schooling

Angrist and Krueger (1991)

Compulsory schooling laws: exogenous variation by quarter of birth

Instrumental variable approach

Local Average Treatment Effect (LATE)

\[ \begin{align} \ln W_{icq} &= \beta X_i + \rho E_i + \sum_c 1\{YOB_i = c\}\xi_c + \mu_i \\ E_{icq} &= \pi X_i + \sum_c 1\{YOB_i = c\}\delta_c + \sum_c\sum_q1\{YOB_i = c\} 1\{QOB_i = q\}\theta_{qc} + \epsilon_i \end{align} \]

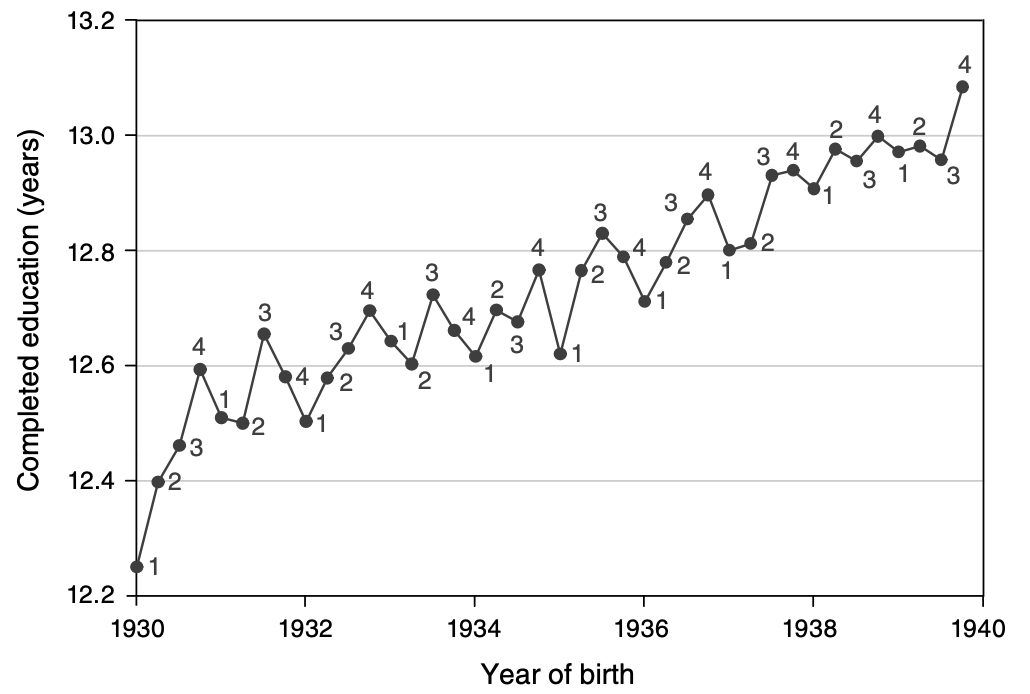
Causal estimates of returns to schooling

Angrist and Krueger (1991)

IV estimates of returns to education \(\rho\)

	1930 cohort	1940 cohort
r	0.076	0.095
	(0.029)	(0.022)
Weak IV F-stat	1.6	3.2

Issues:

• Instrument is weak (IV estimates are inflated)

• Who are the compliers? Endogeneity? External validity?

• Compliers represent only 0.46% of the population (extrapolation issue)

• Compliers are also people that optimally choose min schooling

  • their baseline returns to schooling is likely to be low

  • but because of low base, an exogenous shift in years of schooling can overestimate marginal return to schooling among these people

• Quarter of birth is a very weak instrument => estimates are inflated!

• Trends within cohorts (may not have been fully accounted for by detrending and could reflect changes in the schooling system)

• Endogenous choice of year and quarter of birth by parents

Causal estimates of returns to schooling

Some other IV approaches

	Instrument	Estimated \(\rho\)
Card (1993)	Proximity to college	0.132 (0.055)
Cameron and Taber (2004)	Proximity to college	0.228 (0.109)
Cameron and Taber (2004)	Earnings in local labour market	0.057 (0.115)
Kane and Rouse (1995)	College tuition fees	0.116 (0.045)
Oreopoulos (2007)	Changes in compulsory schooling laws	0.133 (0.0118) US 0.084 (0.0267) Canada 0.158 (0.0491) UK

Causal estimates of returns to schooling

Twin studies

\[ \begin{align} \ln w_{ij} &= \alpha + \rho s_{ij} + A_j + \varepsilon_{ij}, ~\forall i \in \{1, 2\} \\ \Delta \ln w_j &= \rho \Delta s_j + \Delta \varepsilon_j \end{align} \]

	Estimated \(\rho\)
Ashenfelter and Rouse (1998)	0.088 (0.025)
Oreopoulos and Salvanes (2011)	0.0476 (0.0026)

• Variation in schooling between twins \(\Delta s_j\) cannot be correlated with other variables that affect variation in earnings \(\Delta \ln w_j\)

  • This is difficult to justify!

Causal estimates of returns to schooling

Regression discontinuity design: Oreopoulos (2006)

UK 1947: raised min school leaving age (ROSLA) from 14 to 15
Compare similar people just before and after policy change

Estimated \(\rho\) = 0.069 (0.040)

Second reform in 1972: min SLA \(\uparrow\) from 15 to 16
Small (or zero) return (Dickson and Smith 2011)

Source: Figure 1 from Oreopoulos (2006)

Potential questions:

• If returns are so high, why do they not stay up to age 15 to begin with?

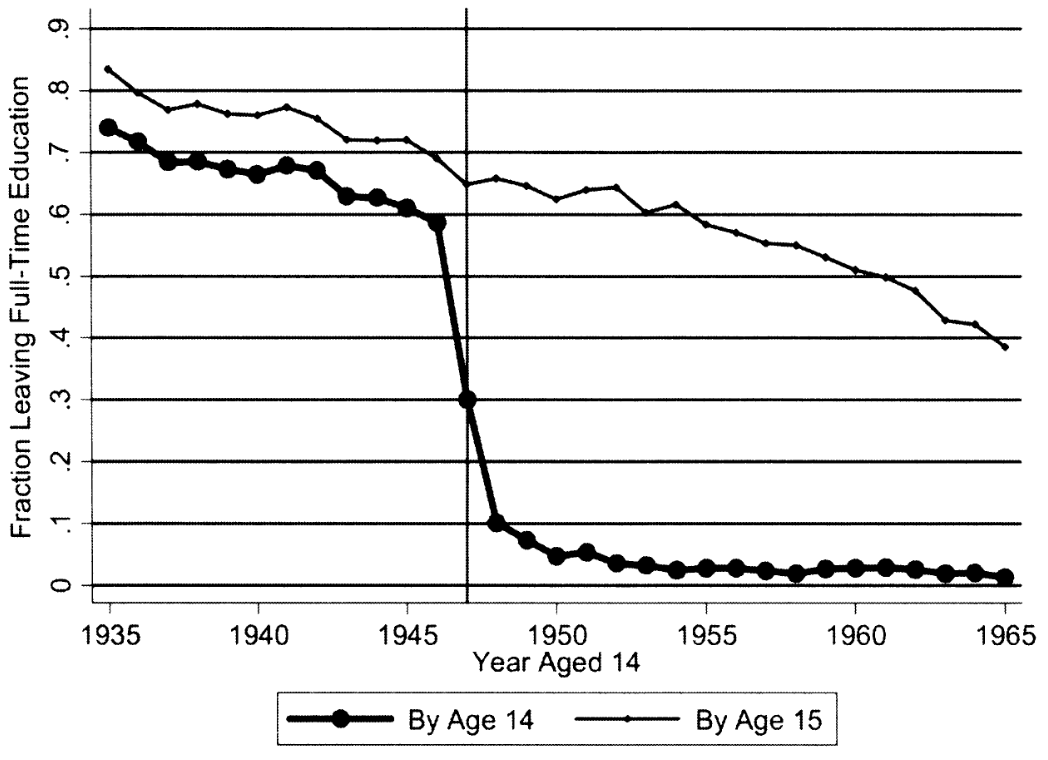
Causal estimates of returns to schooling

Carneiro, Heckman, and Vytlacil (2011)

• Many papers estimate sizable returns to schooling
• Average dropout rate in OECD 17% in 2020
• Heterogeneity in returns to schooling

Role of individual characteristics? E.g., patience (Cadena and Keys 2015)

Source: Carneiro, Heckman, and Vytlacil (2011)

• IV estimates = average of MTE, at best

• Significant selection on gains:

  • dropouts already have very low returns (or negative)

  • those who stay have very high returns to education

• Policy relevant effect thus depends on where along this curve the policy bites

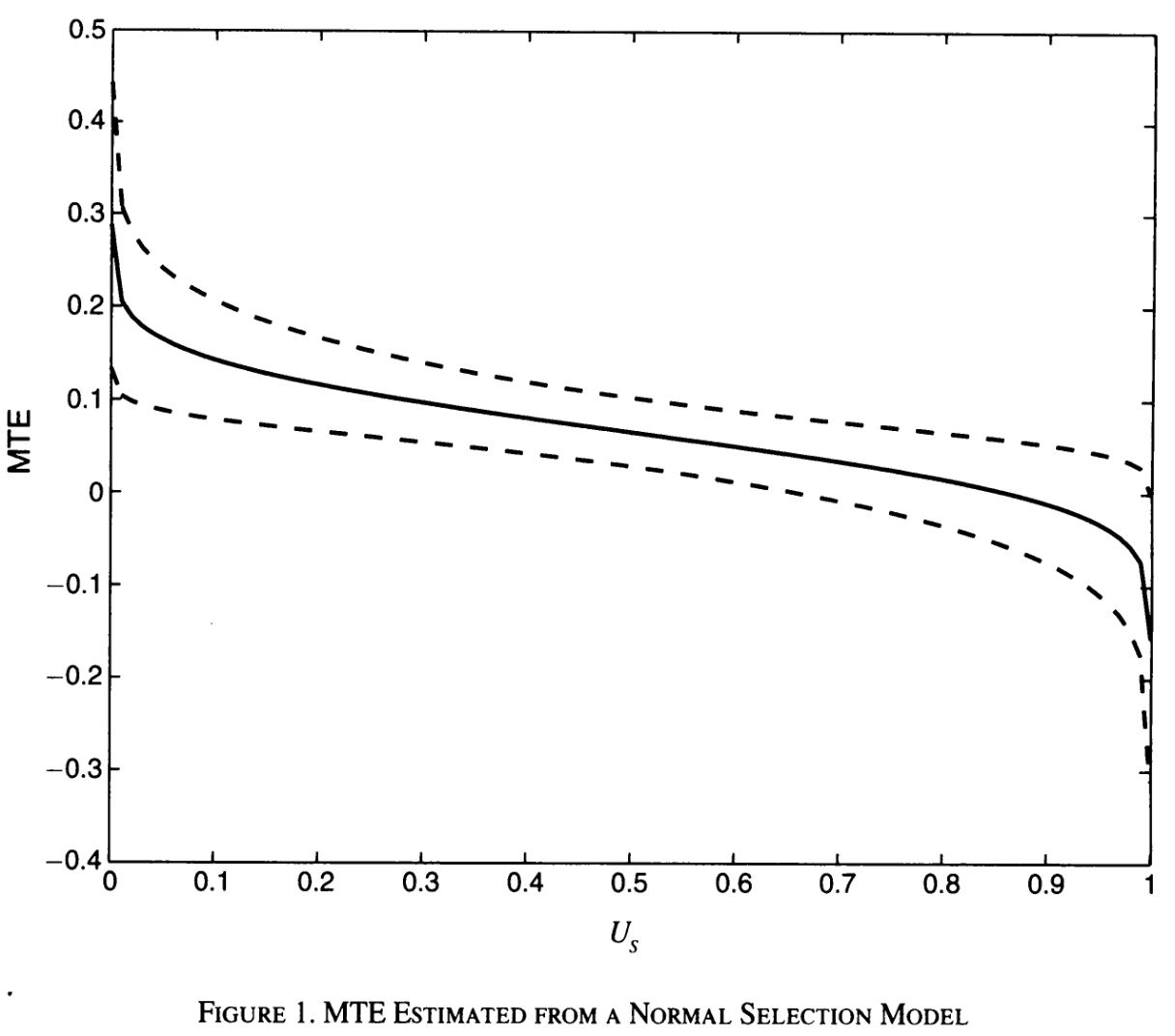
Causal estimates of returns to schooling

Productivity or signalling?

Hard question to answer

Productivity

• [No] upstream effects of ROSLA on qualifications (Chevalier et al. 2004)
• Student riots, \(\uparrow\) passes, \(\uparrow\) higher edu, \(\uparrow\) wages (Maurin and McNally 2008)
• RDD of just passing/failing high school exam: no effect (Clark and Martorell 2014)

Signalling

• Employer learning: 30% of returns due to signalling (Aryal, Bhuller, and Lange 2022)
• Positive effect of degree classes (Feng and Graetz 2017)

Highlight that qualifications have both “productivity” as well as “signalling” parts

Summary

• Education is a human capital investment

• Models describing the investment decisions treat education as productivity enhancing and/or signalling device

• Empirical estimates suggest sizable wage returns to a year of schooling

• However, still a lot of debate about causality, heterogeneity and interpretation

Next: Education Quality

References

Angrist, Joshua D., and Alan B. Krueger. 1991. “Does Compulsory School Attendance Affect Schooling and Earnings?*.” The Quarterly Journal of Economics 106 (4): 979–1014. https://doi.org/10.2307/2937954.

Aryal, Gaurab, Manudeep Bhuller, and Fabian Lange. 2022. “Signaling and Employer Learning with Instruments.” American Economic Review 112 (5): 1669–1702. https://doi.org/10.1257/aer.20200146.

Ashenfelter, Orley, and Cecilia Rouse. 1998. “Income, Schooling, and Ability: Evidence from a New Sample of Identical Twins.” The Quarterly Journal of Economics 113 (1): 253–84. https://www.jstor.org/stable/2586991.

Ben-Porath, Yoram. 1967. “The Production of Human Capital and the Life Cycle of Earnings.” Journal of Political Economy 75 (4): 352–65. https://www.jstor.org/stable/1828596.

Cadena, Brian C., and Benjamin J. Keys. 2015. “Human Capital and the Lifetime Costs of Impatience.” American Economic Journal: Economic Policy 7 (3): 126–53. https://doi.org/10.1257/pol.20130081.

Cahuc, Pierre. 2004. Labor Economics. Cambridge (Mass.): MIT Press.

Cameron, Stephen V., and Christopher Taber. 2004. “Estimation of Educational Borrowing Constraints Using Returns to Schooling.” Journal of Political Economy 112 (1): 132–82. https://doi.org/10.1086/379937.

Card, David. 1993. “Using Geographic Variation in College Proximity to Estimate the Return to Schooling.” NBER Working Paper. Cambridge, MA. October 1993. https://doi.org/10.3386/w4483.

———. 1999. “The Causal Effect of Education on Earnings.” In Handbook of Labor Economics, 3:1801–63. Elsevier. https://doi.org/10.1016/S1573-4463(99)03011-4.

Carneiro, Pedro, James J. Heckman, and Edward J. Vytlacil. 2011. “Estimating Marginal Returns to Education.” The American Economic Review 101 (6): 2754–81. https://www.jstor.org/stable/23045657.

Chevalier, Arnaud, Colm Harmon, Ian Walker, and Yu Zhu. 2004. “Does Education Raise Productivity, or Just Reflect It?” The Economic Journal 114 (499): F499–517. https://www.jstor.org/stable/3590169.

Clark, Damon, and Paco Martorell. 2014. “The Signaling Value of a High School Diploma.” Journal of Political Economy 122 (2): 282–318. https://doi.org/10.1086/675238.

Cunha, Flavio, and James Heckman. 2007. “The Technology of Skill Formation.” American Economic Review 97 (2): 31–47. https://doi.org/10.1257/aer.97.2.31.

Dickson, Matt, and Sarah Smith. 2011. “What Determines the Return to Education: An Extra Year or a Hurdle Cleared?” Economics of Education Review, Special Issue: Economic Returns to Education, 30 (6): 1167–76. https://doi.org/10.1016/j.econedurev.2011.05.004.

Feng, Andy, and Georg Graetz. 2017. “A Question of Degree: The Effects of Degree Class on Labor Market Outcomes.” Economics of Education Review 61 (December): 140–61. https://doi.org/10.1016/j.econedurev.2017.07.003.

Heckman, James, Jora Stixrud, and Sergio Urzua. 2006. “The Effects of Cognitive and Noncognitive Abilities on Labor Market Outcomes and Social Behavior.” Journal of Labor Economics 24 (3): 411–82.

Kane, Thomas J., and Cecilia Elena Rouse. 1995. “Labor-Market Returns to Two- and Four-Year College.” The American Economic Review 85 (3): 600–614. https://www.jstor.org/stable/2118190.

Maurin, Eric, and Sandra McNally. 2008. “Vive La Révolution! Long‐Term Educational Returns of 1968 to the Angry Students.” Journal of Labor Economics 26 (1): 1–33. https://doi.org/10.1086/522071.

Mincer, Jacob. 1958. “Investment in Human Capital and Personal Income Distribution.” Journal of Political Economy 66 (4): 281–302. https://www.jstor.org/stable/1827422.

Mincer, Jacob A. 1974. Schooling, Experience, and Earnings. Book. National Bureau of Economic Research. https://www.nber.org/books-and-chapters/schooling-experience-and-earnings.

Oreopoulos, Philip. 2006. “Estimating Average and Local Average Treatment Effects of Education When Compulsory Schooling Laws Really Matter.” American Economic Review 96 (1): 152–75. https://doi.org/10.1257/000282806776157641.

———. 2007. “Do Dropouts Drop Out Too Soon? Wealth, Health and Happiness from Compulsory Schooling.” Journal of Public Economics 91 (11-12): 2213–29. https://doi.org/10.1016/j.jpubeco.2007.02.002.

Oreopoulos, Philip, and Kjell G Salvanes. 2011. “Priceless: The Nonpecuniary Benefits of Schooling.” Journal of Economic Perspectives 25 (1): 159–84. https://doi.org/10.1257/jep.25.1.159.